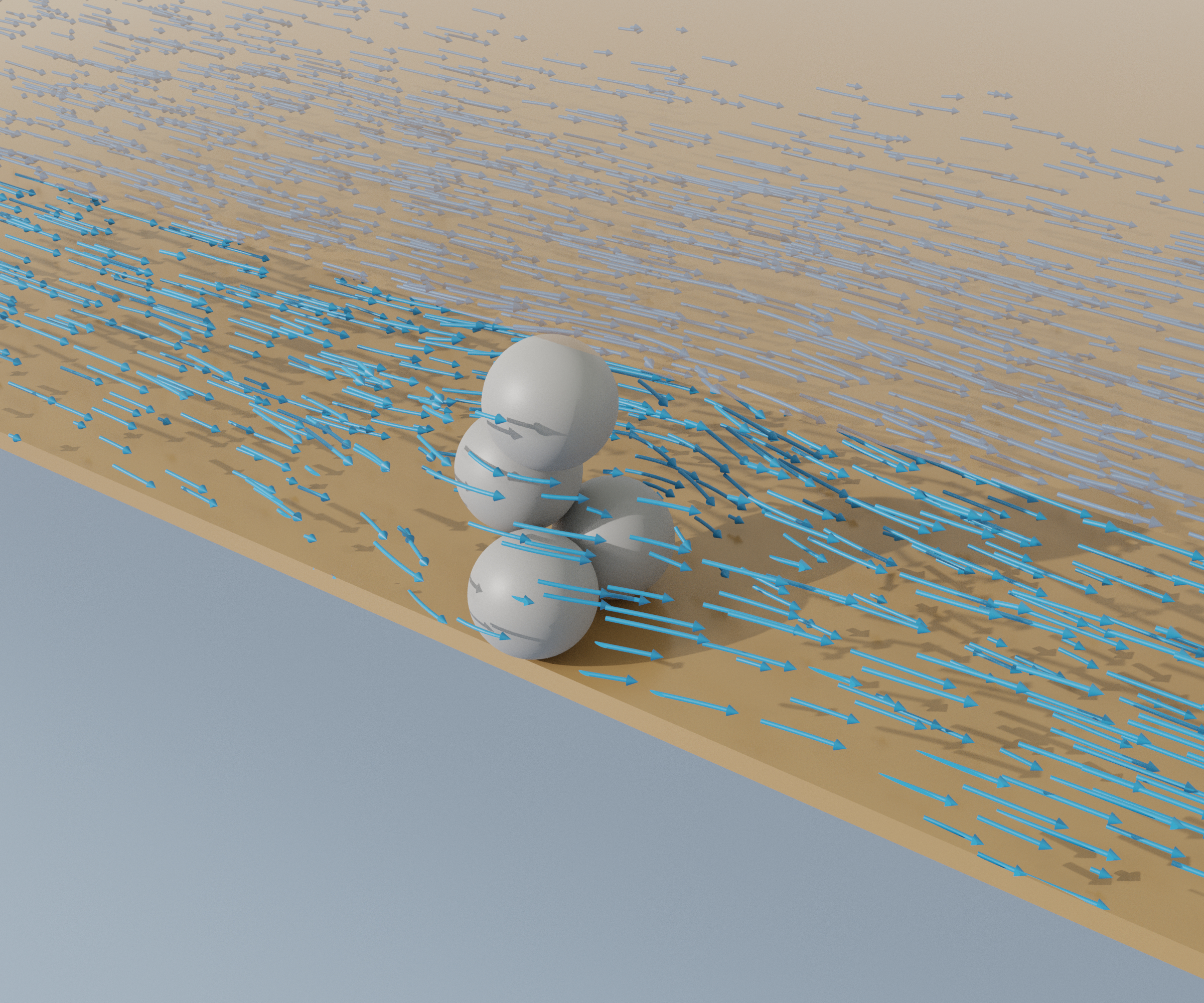
Hydrodynamic clogging of micro-particles in planar channels under electrostatic forces
Particle clogging can occur in any scenario where a flow path or constriction is small relative to the size of objects trying to pass through it - think of flow through silos, blood flows through needles, and even the evacuation of crowds through barrieres. It originates from the formation of a single arch, behind which an entire flow path can become blocked. This work is the first time hydrodynamic clogging has been thoroughly investigated in planar channels.
Quantifying the Permeability Enhancement from Blast-Induced Microfractures in Porphyry Rocks Using a Cumulant Lattice Boltzmann Method
Abstract The permeability of rocks is important in a range of geoscientific applications, including CO 2 sequestration, geothermal energy extraction, and in situ mineral recovery. This work presents an investigation of the change in permeability in porphyry rock samples due to blast-induced fracturing. Two samples were analysed before and after exposure to stress waves induced by the detonation of an explosive charge. Micro-computed tomography was used to image the interior of the samples at a pixel resolution of 10.
A comparative study of 3D cumulant and central moments lattice Boltzmann schemes with interpolated boundary conditions for the simulation of thermal flows in high Prandtl number regime
Abstract Thermal flows characterized by high Prandtl number are numerically challenging as the transfer of momentum and heat occurs at different time scales. To account for very low thermal conductivity and obey the Courant-Friedrichs-Lewy condition, the numerical diffusion of the scheme has to be reduced. As a consequence, the numerical artefacts are dominated by the dispersion errors commonly known as wiggles. In this study, we explore possible remedies for these issues in the framework of lattice Boltzmann method by means of applying novel collision kernels, lattices with large number of discrete velocities, namely D3Q27, and a second-order boundary conditions.
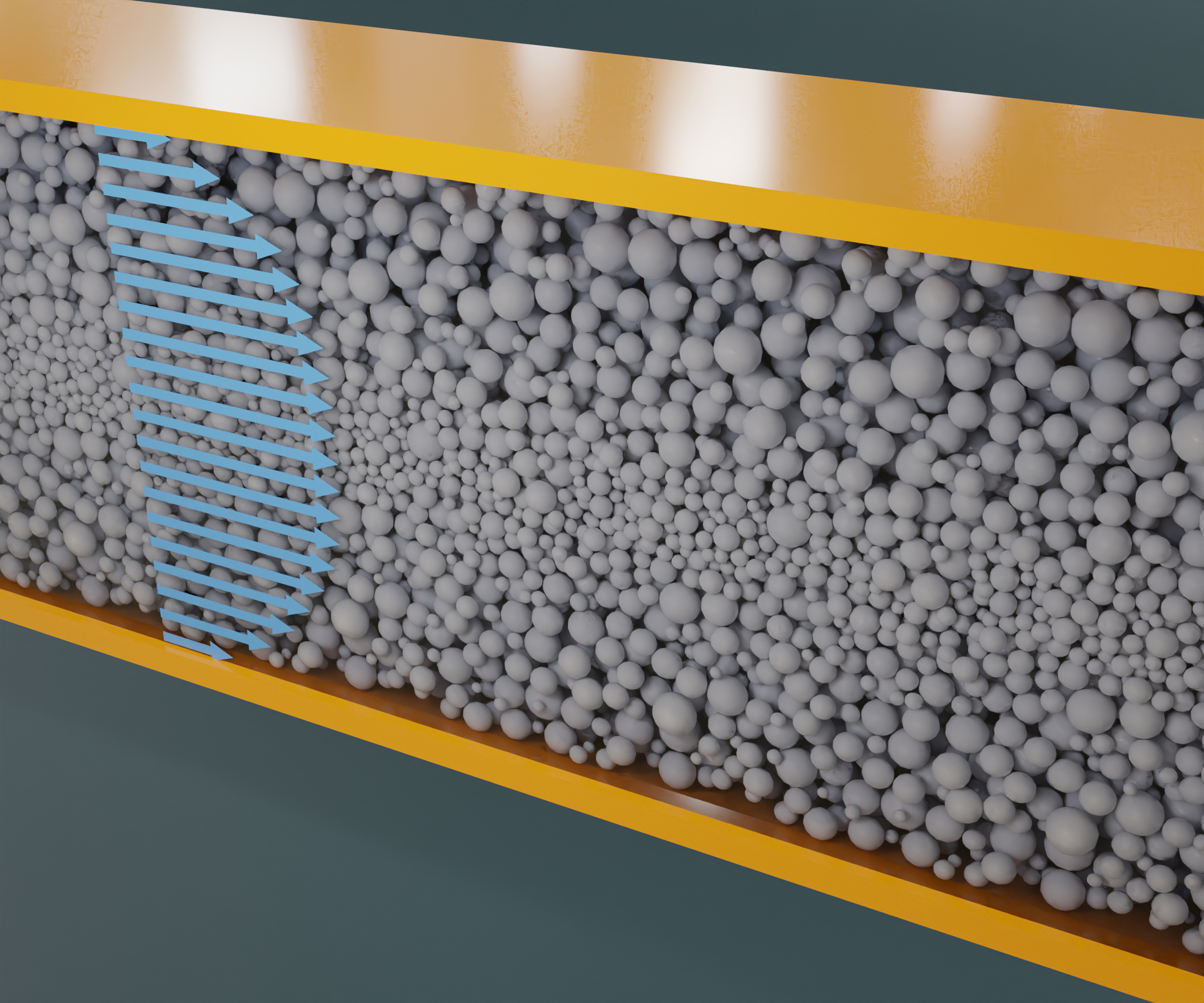
Influence of particle polydispersity on bulk migration and size segregation in channel flows
In this work we investigated the phenomenon of shear-induced migration for polydisperse suspensions for the first time. Shear-induced migration is basically the diffusion of particles in the direction of decreasing shear rate, caused by the accumlation of random particle collisions in sheared flows. In channels, it results in the accumulation of particles at the channel centre and a flattening of the velocity profile. These concepts have long been established and investigated for monodisperse suspensions (all particles of the same size), and some works have even investigated bidisperse suspensions (particles of two different sizes), demonstrating that the larger particles preferentially migrate towards the channel centre.
Memory-efficient Lattice Boltzmann Method for low Reynolds number flows
Abstract The Lattice Boltzmann Method algorithm is simplified by assuming constant numerical viscosity (the relaxation time is fixed at τ=1). This leads to the removal of the distribution function from the computer memory. To test the solver the Poiseuille and Driven Cavity flows are simulated and analyzed. The error of the solution decreases with the grid size L as L−2. Compared to the standard algorithm, the presented formulation is simpler and shorter in implementation.
A 3D LBM-DEM study of sheared particle suspensions under the influence of temperature-dependent viscosity
Abstract Particle suspensions form a fundamental yet complex component of many scientific and engineering endeavours. This paper proposes a numerical coupling between the lattice Boltzmann and discrete element methods that resolves particle suspensions exposed to thermal influences due to temperature-dependent fluid viscosity and conjugate heat transfer between components. Validation of the model was performed via the study of the relative viscosity of suspensions. This numerically corroborated the proposed temperature-dependence of the relative viscosity of suspensions.
Lattice Boltzmann simulation of transient blood flow in arterial geometries using a regularised, viscoplastic and shear-thinning fluid
Abstract This paper presents a lattice Boltzmann framework for the transient simulation of blood flow using biologically inspired geometries and pressure boundary conditions. The Kuang-Luo rheological model is used to represent blood as a homogeneous continuum. This model includes the two primary non-Newtonian characteristics of blood, namely viscoplasticity and pseudoplasticity. This paper makes two contributions. First, the numerical challenges associated with zero strain rates and infinite viscosity, as a consequence of the yield stress in the Kuang-Luo model, were addressed by regularising the constitutive equation so that the viscosity tends towards a finite value at low strain rates.
Numerical investigation of the effects of proppant embedment on fracture permeability and well production in Queensland coal seam gas reservoirs
Abstract This paper introduces the development of a new predictive model in support of proppant injection in naturally fractured coal seam gas (CSG) reservoirs. In the proposed model, the finite element method (FEM) is used for the prediction of proppant embedment and elastoplastic deformation of the coal. The lattice Boltzmann method (LBM) is applied to the modelling of fluid flow through propped fractures, in which the modified partially saturated method (MPSM) is implemented to characterise the fluid–solid interactions.
Computational modeling of three-dimensional thermocapillary flow of recalcitrant bubbles using a coupled lattice Boltzmann-finite difference method
Abstract This study analyzes the thermocapillary flow of recalcitrant bubbles within thin channels using a hybrid finite difference lattice Boltzmann method (LBM). It extends a recently developed phase-field LBM to account for temperature effects by coupling the scheme with a fourth-order Runge-Kutta algorithm to solve the governing energy equation. The LBM makes use of a weighted-multiple relaxation-time collision scheme, which has been previously shown to capture high density and viscosity contrasts.
On the rise characteristics of Taylor bubbles in annular piping
Abstract A three-dimensional phase-field lattice Boltzmann method has been applied to investigate the rise of Taylor bubbles within annular pipes. The approach couples the conservative phase-field model with a velocity-based lattice Boltzmann scheme. The implementation uses 27 discrete velocities to resolve both the interfacial dynamics and the hydrodynamics. To assist numerical stability for the high-density ratio, two-phase flows a weighted multiple-relaxation-time collision operator is employed. This paper makes contributions in three areas.
Open-loop optimal control of a flapping wing using an adjoint Lattice Boltzmann method
Abstract We present the usage of an adjoint Lattice Boltzmann Method (LBM) for open-loop control of two-dimensional flapping wing motion. Movement of the wing is parametrised with periodic B-Splines, while the wing interacts with the surrounding flow via an Immersed Boundary (IB) method. Multi-objective optimisation is performed using a gradient optimisation algorithm, for which sensitivities are calculated with an adjoint method. The objectives selected were the mean lift force and mechanical power.
Development of closure relations for the motion of Taylor bubbles in vertical and inclined annular pipes using high-fidelity numerical modeling
Abstract This study analyses the flow of Taylor bubbles through vertical and inclined annular pipes using high-fidelity numerical modeling. A recently developed phase-field lattice Boltzmann method is employed for the investigation. This approach resolves the two-phase flow behavior by coupling the conservative Allen-Cahn equation to the Navier-Stokes hydrodynamics. This paper makes contributions in three fundamental areas relating to the flow of Taylor bubbles. First, the model is used to determine the relationship between the dimensionless parameters (Eötvös and Morton numbers) and the bubble rise velocity (Froude number).
Transport of particles suspended within a temperature-dependent viscosity fluid using coupled LBM–DEM
Abstract Particle suspensions are present in a wide variety of practical settings. Modelling these numerically is a challenging task that often requires the combination of multiple methodologies. This paper examines particle transport within a temperature-dependent viscosity fluid utilising a coupled approach of the lattice Boltzmann method and the discrete element method. This technique takes advantage of the locality of the lattice Boltzmann method to allow both the individual particle behaviour to be fully resolved and to permit fine-scale variation of fluid viscosity throughout the tested domains.
A cascaded phase-field lattice Boltzmann model for the simulation of incompressible, immiscible fluids with high density contrast
Abstract In this work, a conservative phase-field model for the simulation of immiscible multiphase flows is developed using an incompressible, velocity-based, cascaded lattice Boltzmann method (CLBM). Extensions are made to the lattice Boltzmann (LB) equations for interface tracking and incompressible hydrodynamics, proposed by Fakhari et al. [1], by performing relaxation operations in central moment space. This was motivated by the work of Fei et al. [2,3], where promising results from such a transformation were observed.
Investigation of local and non-local lattice Boltzmann models for transient heat transfer between non-stationary, disparate media
Abstract Modelling the thermodynamic and hydrodynamic interactions of suspended particles is a significant and ongoing numerical challenge. Addressing this is necessary in order to be able to fully model numerous industrial and scientific processes of practical interest. This paper describes extensions to a local and a non-local technique for the calculation of transient conjugate heat transfer within a lattice Boltzmann framework. The interface transition between phases in both methods has been incorporated via a partially saturated boundary condition that weights material properties and allows straight and curved boundaries to be captured.
Development of a three-dimensional phase-field lattice Boltzmann method for the study of immiscible fluids at high density ratios
Abstract Based on the recent work by Fakhari et al. (2017b), a three-dimensional phase-field lattice Boltzmann method was developed to investigate the rise of a Taylor bubble in a duct. The proposed approach couples the conservative phase-field equation with a velocity-based lattice Boltzmann scheme equipped with a weighted multiple-relaxation-time collision operator to enhance numerical stability. This makes the model ideal for numerical simulation of immiscible fluids at high density ratios and relatively high Reynolds numbers.
Depth-averaged Lattice Boltzmann and Finite Element methods for single-phase flows in fractures with obstacles
Abstract We use Lattice Boltzmann Method (LBM) MRT and Cumulant schemes to study the performance and accuracy of single-phase flow modeling for propped fractures. The simulations are run using both the two- and three-dimensional Stokes equations, and a 2.5D Stokes–Brinkman approximate model. The LBM results are validated against Finite Element Method (FEM) simulations and an analytical solution to the Stokes–Brinkman flow around an isolated circular obstacle. Both LBM and FEM 2.
Single component multiphase lattice boltzmann method for taylor/bretherton bubble train flow simulations
Abstract In this study long bubble rising in a narrow channel was investigated using multiphase lattice Boltzmann method. The problem is known as a Bretherton or Taylor bubble flow [2] and is used here to verify the performance of the scheme proposed by [13]. The scheme is modified by incorporation of multiple relaxation time (MRT) collision scheme according to the original suggestion of the author. The purpose is to improve the stability of the method.
Adjoint Lattice Boltzmann for topology optimization on multi-GPU architecture
Abstract In this paper we present a topology optimization technique applicable to a broad range of flow design problems. We propose also a discrete adjoint formulation effective for a wide class of Lattice Boltzmann Methods (LBM). This adjoint formulation is used to calculate sensitivity of the LBM solution to several type of parameters, both global and local. The numerical scheme for solving the adjoint problem has many properties of the original system, including locality and explicit time-stepping.
Pressure drop in flow across ceramic foams-A numerical and experimental study
Abstract The unique properties of ceramic foams make them well suited to a range of applications in science and engineering such as heat transfer, reaction catalysis, flow stabilization, and filtration. Consequently, a detailed understanding of the transport properties (i.e. permeability, pressure drop) of these foams is essential. This paper presents the results of both numerical and experimental investigations of the morphology and pressure drop in 10. ppi (pores per inch), 20.